Schedule:

9:00-11:00, Monday 13/06 through Friday 17/06.

 

Abstract:

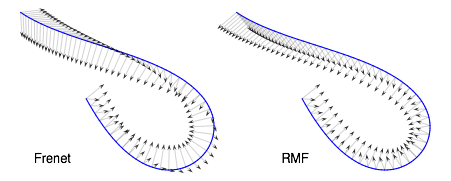
It is impossible to parameterize any curve, other than a straight line, by rational functions of its arc length. Nevertheless, the polynomial Pythagorean hodograph (PH) curves offer progress toward this impossible ideal by incorporating a special algebraic structure, which ensures that the arc length is simply a polynomial in the curve parameter. To achieve a rotation-invariant formulation in the case of spatial PH curves, this structure is expressed as a quaternion product. This course reviews the

quaternion formulation of spatial PH curves, their fundamental properties, their construction through the interpolation of discrete geometrical data, their applications in real-time motion control, and criteria for the existence of rational rotation-minimizing frames.

 

Slides and notes: